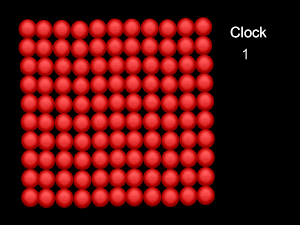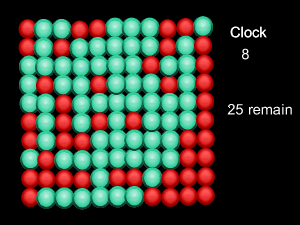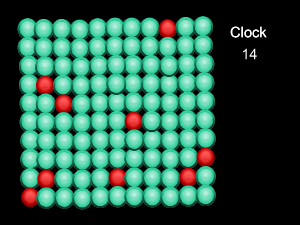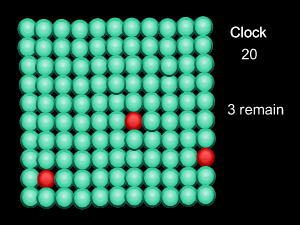
Radioactive isotopes decay at different rates. The rate of decay is measured in terms of the half-life. Hlaf-life is the time needed for half of the radioactive material to decay and become one or more different elements.
The isotope radium-226 has a half life of 1620 years. That is half of any given quantity of 226Ra will have decayed by the end of 1620 years.
The half-life of the 238U isotope is 4.5 billion years.
The rate of decay of radioactive isotopes appears to be constant unaffected by external factors such as tremperature and magnetic fields.
Isotopes with a shorter half-life tend to decay quicker.
A 400 gram sample of radioactive isotope "X" is placed in a container and left for three years. If its half-life is 1 year what mass of X is present after three years?
The half-life of two radioactive isotopes X and Y are 12 years and 1 year respectively. If equal quantites of both isotopes are measured which one of the following comments is therefore true?
A pure sample of a radioactive isotope X contains 100 atoms. If its half-life is 4 years how much is present after 12 years? The solution is shown on the right.
If a 1,000 gram sample of this isotope is allowed to stand for 5 years what mass of this isotope will remain?